Secrets of fibonacci series

The Fibonacci numbers are Nature's numbering system. They appear everywhere in Nature, from the leaf arrangement in plants, to the pattern of the florets of a flower, the bracts of a pinecone, or the scales of a pineapple. The Fibonacci numbers are therefore applicable to the growth of every living thing, including a single cell, a grain of wheat, a hive of bees, and even all of mankind.

Plants do not know about this sequence - they just grow in the most efficient ways. Many plants show the Fibonacci numbers in the arrangement of the leaves around the stem. Some pine cones and fir cones also show the numbers, as do daisies and sunflowers. Sunflowers can contain the number 89, or even 144. Many other plants, such as succulents, also show the numbers. Some coniferous trees show these numbers in the bumps on their trunks. And palm trees show the numbers in the rings on their trunks.

Why do these arrangements occur? In the case of leaf arrangement, or phyllotaxis, some of the cases may be related to maximizing the space for each leaf, or the average amount of light falling on each one. Even a tiny advantage would come to dominate, over many generations. In the case of close-packed leaves in cabbages and succulents the correct arrangement may be crucial for availability of space.

In the seeming randomness of the natural world, we can find many instances of mathematical order involving the Fibonacci numbers themselves and the closely related "Golden" elements.

Fibonacci in Plants

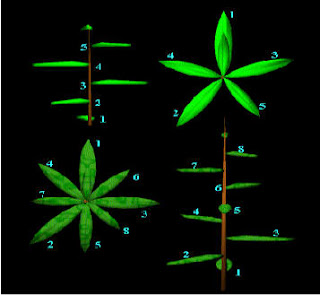
 Phyllotaxis is the study of the ordered position of leaves on a stem. The leaves on this plant are staggered in a spiral pattern to permit optimum exposure to sunlight. If we apply the Golden Ratio to a circle we can see how it is that this plant exhibits Fibonacci qualities. Click on the picture to see a more detailed illustration of leaf arrangements.

                                                                       

By dividing a circle into Golden proportions, where the ratio of the arc length are equal to the Golden Ratio, we find the angle of the arcs to be 137.5 degrees. In fact, this is the angle at which adjacent leaves are positioned around the stem. This phenomenon is observed in many types of plants.

                                                         

In the case of tapered pinecones or pineapples, we see a double set of spirals – one going in a clockwise direction and one in the opposite direction. When these spirals are counted, the two sets are found to be adjacent Fibonacci numbers. 

Similarly, sunflowers have a Golden Spiral seed arrangement. This provides a biological advantage because it maximizes the number of seeds that can be packed into a seed head.

  

Inside the fruit of many plants we can observe the presence of Fibonacci order.

     

                                                                                       

        

         The banana has 3 sections     The apple has 5 sections

As well, many flowers have a Fibonacci number of petals. Some, like this rose, also have Fibonacci, or Golden Spiral, petal arrangements.

 Branching plants also exhibit Fibonacci numbers. Again, this design provides the best physical accommodation for the number of branches, while maximizing sun exposure.

 Fibonacci Petals

3 petals                  lily, iris

5 petals                  buttercup, wild rose, larkspur, columbine

8 petals                 delphiniums

13 petals                ragwort, corn marigold, cineraria

21 petals                aster, black-eyed susan, chicory

34 petals                plantain, pytethrum

55, 89 petals          michelmas daisies, the asteraceae family

The occurrence of Fibonacci Numbers in Nature is interesting but the ratio of consecutive Fibonacci Numbers is important.

Fibonacci in Animals

The shell of the chambered Nautilus has Golden proportions. It is a logarithmic spiral.

The eyes, fins and tail of the dolphin fall at Golden sections along the body.

A starfish has 5 arms.  (5 is the 5th Fibonacci number)

If a regular pentagon is drawn and diagonals are added, a five-sided star or pentagram is formed. Where the sides of the pentagon are one unit in length, the ratio between the diagonals and the sides is Phi, or the Golden Ratio. This five-point symmetry with Golden proportions is found in starfish.

Humans exhibit Fibonacci characteristics, too. The Golden Ratio is seen in the proportions in the sections of a finger.

 

It is also worthwhile to mention that we have 8 fingers in total, 5 digits on each hand, 3 bones in each finger, 2 bones in 1 thumb, and 1 thumb on each hand.

The ratio between the forearm and the hand is the Golden Ratio!

The cochlea of the inner ear forms a Golden Spiral.

The question must arise as to why the golden proportion is special - and more importantly, is there any difference between the Golden Proportion and another pleasing proportion? A brief study of figures below will answer this question.

Thus the proportion of the smaller to the greater is the same as the proportion of the greater to the whole. The division of the line by point C thus represents a point of equilibrium between these two proportions. If you move the point a fraction one way or the other, then you have two proportions which are neither the same nor are they in equilibrium. The only time that these two proportions are the same is when they are Golden.

This point of division is a mathematical confirmation of how the eye senses the balance of this magical proportion that appears so frequently in nature and art.